Work Period

These are things you shoud probably work on today

1.) Stats portion of our formula sheet for tuesdays test.

2.) Applications of periodic functions portion of tuesdays test.

3.) These questions from the handout for tomorrow : 3, 10, 16.

4.) Accelerated math (Stats and periodic function objectives).

Exam information

Exam is Wednesday June 13 at 9:00 AM to 11:00 AM and Thursday June 14 at 9:00 AM to 11:30 AM. The first day of the exam will be two questions, worth 25% of the exam mark and the second day is the written part of the exam worth roughly 75% of your exam mark.

Hopewell Rocks

Yesterday, Mr.Max left class for a little while, so we had time to do our homework. Upon his return, we started to correct our homework; which is displayed below.

The first question asks: "what is the period of this ferris wheel?"

Using the formula period = 2╥/b, in this case it is:

Period = 2╥/0.523

if you put this into your calculator, then you will get 12.01. Do not round it off to 12, because Senior 4 Applied has to be accurate to 2 decimal places.

The second question asks: "What does that mean (in this question)"

This simply means that Michelle's ferris wheel makes one complete revolution (rotation, spin, turn, go around) in 12.01 minutes.

The third question asks: "After 4 minutes, how high is a person on the wheel? (State ANY assumptions"

There are many different answers you could have for this question, but you will only get marks if you show what you were thinking, or any assumptions you make that would change the answer

Some people may assume that you get on the ferris wheel at its lowest point, some may assume that you get on the ferris wheel when the graph line crosses the y-axis, along with many other assumptions.

The mathematically correct answer is 4.94, but if you assume otherwise, then the answer will change correspondingly.

And finally the last question states, "make the ferris wheel more fun (faster)" This can be done by changing x, Mr.Max will not accept an answer like:

y=22sin(100000000000x-3.14)+24

This is unrealistic because if you were going that fast you would be thrown of from the insane amount G-force

You could make it something like:

y=22sin(10x-3.14)+24

We then started Sinusoidal Regression

We went to this web site here, and found a prediction for the Hopewell Rocks

You can load this data into your calculator,

STAT, enter,


Then hit STAT, over to CALC, up one to SinReg, enter, L1, L2, (unless you put them in different columns) Y1,


(Y1 is found by hitting VARS, over to Y-VARS, enter, enter)


it then displays a,b,c, and d


Then we looked up Hopewell Rocks on Google Earth, and that was the end of math that day because we just kept looking up different places like, Mr.Max's brother's house in Calgary, a picnic table, that Mr.Max had supper on. Also looking at people that were walking on the sidewalk, or driving there cars.


I thought it was quite incredible how humans have made such remarkable technology, so sufisticaed, that you can see people walking from space. I think it would be cool if it were live video... but you would need a pretty crazy computer probably.



Also, this is completely random, but a boy shot a boar, (a male wild pig) with a pistol a little while ago in Alabama, it was over 1,000 pounds, and 9 feet 4 inches long!! Click here to see a web site about it.




Well, thats it, have a nice day!

ABCD Values

Today we started off with a homework check...and thats right, you guessed it....only 2 people actually did their homework. Mr. Max was so proud. He was actually quite disappointed since the 2 people who did their homework said it only took them like 5 minutes. That was the 2nd "homework speech" Mr. Max has had to give our class in only about a week's time. Do we want to graduate? I think we all want to, no question about it. So I guess a little extra effort is in order.

Mr. Max said the only equation we need to know is y=a*sin(bx+c)+d. So he started to teach us what a,b,c, and d mean. He referenced what we learned last week, about what happens when the values of a,b,c, and d change. Then he went right into teaching us about "a".

Many people were confused with the whole "a" is amplitude but "a" is not equal to amplitude. So Mr. Max changed the notes to say that "a" is closely related to amplitude and the absolute value of "a" is equal to amplitude. Hope that makes more sense.

Mr. Max moved on to the "b" value. This time Mr. Max made the notes clear and stated that the "b" value relates very closely with period. Although period does not equal the "b" value.

Next, came the "c" value. This relates very closely with horizontal shift or phase shift.

Last,but not least, is the "d" value. This is the vertical shift. It is always positive.

And then, once again Mr. Max assigned homework. Mostly only because he was extremely (I was going to put that into capital letters, but I didn't know if it would be appropriate to shout..) unhappy with us. This is the question Mr. Max came up with :

SVRSS Math 40SA May 25 2007

Hello everyone, we started out the class without Mental Math but correcting the assignment he assigned the day before. We made corrections and helped us out with any problems we had with those questions.

We then learned about a new website on how to figure out Trigonometric functions: www.mathdemos.gesu.edu/mathdemos/sinusoidapp/sinusoidapp.html

So the homework for the weekend is that we had to make our own notes from a Winnipeg blog about the formula y=asin(bx+c)+d. Enjoy and have a good weekend...

Confidence Intervals

Hi.
This is Wilson scribing again.
Some people might be missing the first half of next week for the Provincial English Language Arts Exam. I write the exam in the morning, so it does not actually affect this math course.
Enough about that, now to some work.
Today we learned about Confidence Intervals “Predicting the mean of a sample”
The main idea is to be able to predict with varying level of accuracy (Confidences) the mean of a sample.
Usually levels of confidence are measured at 90% 95% and 99%.
Mr. Maxx’s nice diagrams might show it even better than I could tell you though.

I hope this helps you (all you viewers out there in the electronic world) to understand.

Attempting the Unthinkable

Today Mr. Max attempted the alleged impossible. He undertook a intense mathematic mission, trying to complete the statistics unit in record time. He informed us that if all went well, statistics would be no more after today, May 23, 2007. I know this will not be an easy task; he will need all the help he can get. Luckily he has his partner, Ron Blonde, to lend a helping hand. Ron will be a major part in helping him finish off ‘Binomial Distributions’

Let the mission begin.



Binomial Distribution exists in all scenarios. It includes exactly two outcomes, SUCCESS and FAILURE, which means not success.

Things to remember:
p + q = 1
q = 1 – p
p = 1 – q
q = p!
p = q!

binomcdf formula: ( number of trials, probability of success, number of successes)
binompdf formula: ( number of trials, probability of success, number of successes)

The binomcdf formula is used up to and including this many successes.
The binompdf formula is exactly this many successes.

So after learning all of this information, we tried a couple of practice questions.


The probability that a student at Brandon University uses a particular brand of toothbrush is 2/5. If 6 students selected at random, find the probability that:


A) Exactly three of them use the toothbrush





B) All of them use the toothbrush


C) None of them use the toothbrush


Mr. Max showed us a few ways to do these questions, using our calculator or using his partner Ron's website.


As 2 o'clock rolled around Mr. Max informed us that mission was accomplished. ...NOT!! We still have one more day left in this unit, finishing off Confidence Intervals tomorrow. Our test will still be on the same day, June 5th.

Stats

Sorry this post is late, I was not around a computer all weekend. We had no homework for the weekend, but our class started off by Mr. Max asking us to put the answers for 8, 12, and 15 on the blog. After he gave us a little time to do that, he started going through them.






















And those are the answers. Just a reminder that our test for stats and periodic functions is on June 5th.

Question #15

Well, how do you do it? What's the answer? Why? How can you justify your answer? Comment Below?

Question #12

Well, how do you do it? What's the answer? Why? How can you justify your answer? Comment Below?

Question #8

Well, how do you do it? What's the answer? Why? How can you justify your answer? Comment Below?

Using Words Rather Than Colors

So, once again I am the scribe by some strange(dumb?) luck. Today was our first day of the rest of the semester without mental math. We began the class with Mr. Max going over question # 1. of the Statistics Review booklet. We were supposed to do the question in each of the four ways we were taught yesterday to do it. So Mr. Max went over the question using each method.

1.)

2.)

3.)

* ronblond's site does not accept thousandths rounding (ie: 0.013) therefore, plan accordingly!

* enter the boundaries into the same Letter not Color.

4.)

Z-Scores and Standardized Scores:

"A z-score is simply a value created in conjunction with a data sets mean and standard deviation to determine a value's number of standard deviations from the mean."

* Positive z-score: ABOVE the mean
*Negative z-score: BELOW the mean

Mr. Max also talked about the U.F.E exam.

Finally, at the end of the class we were given an assignment:

Infinity is represented on the TI-83/84+ as Egg

Today was the last day of mental math, ever {Ever[like, ever(it will be no more in this class)]} and so Mr. Max was very...sombre about it. So to commemorate and truly honor the last day of mental math, it was extra hard...

Then we were given a speech on how "the Internet is forever". He makes a very valid point, if you put something online (this post for example) it will be online forever. Yes you can delete it and take it off the web but people, somehow, will still have access to it.
This is a commercial for Mr. Max's speech. It really makes you think, and be nervous. Mr. Max also told us that if we get bored while he's teaching to take a look at the 3rd block classes blog.

Anyways, after the speech and telling us to look at the block 3 class blog, Mr. Max began to go over the question he assigned yesterday. I don't know about everyone else but I was lost on that question.




Today Mr. Max showed us four ways that we can solve questions involving Z scores.
(Z score: a measure that quantifies the distance a data point is from the mean of a data set.
basically,a measure of how far from the average of a set of data a number is)

1.) normalpdf(x) and shadenorm(Lowest number, Highest number)

* Infinity on the TI-83/84+ is represented as E99
-> To get this press:

2.) y=normalcdf(Low Z score, High Z score)

3.) www.ronblond.com/M12/norm.big.APPLET/index.html

4.) He also put a spreadsheet into our folder on the course work drive, get there by going to:
coursework, Max's math stuff version 2, 2nd semester block 4 applied40, variability and statistical analysis, STATISTICS

Then, at the end of the class Mr. Max assigned the question about chicken freshness or something...

Normal Distributions

Today we started out with mental math, and Mr. Max told us that tomorrow will be our last mental math quiz this year. Today couldn't be the last one because Mr. Max couldn't face ending with the number 34, the number has to end in a 5 or a 0. I'm not going to comment on that.....

Mr. Max checked homework from Thursday and he was quite surprised that our sub loved us. Mr. Max, we can't help that we're amazing.

The focus today was on normal distributions, and although we didn't really do any math, Mr. Max assured us that all the rules we have been learning will help us out when we finally get to do some problems.





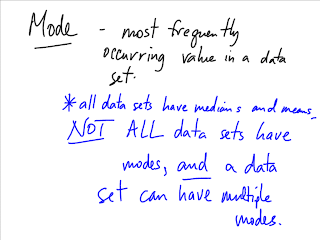
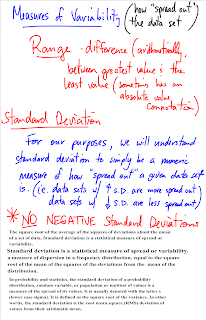
Mr. Max tried to trick us by asking if we knew what a frequency polygon was. I really didn't know what he was talking about, so he then asked if we knew what a frequency histogram was, since they are the same thing. While in all reality he could have just said it was a simple bar graph, because then everybody would understand. Tricky, tricky.

Mr. Max referenced the site we were to work on yesterday. He showed us many of the different gadgets on the site that had something to do with normal distributions.

We took some notes and Mr. Max taught us about the bell curve and some distribution characteristics. He also made the lovely diagram above, which turned out quite nicely I might add, to show us the meanings of the characteristics. He showed us the 68-95-99.7 rule. Which didn't really make sense when you added up the numbers in the graph, but Mr. Max assured us "he just knew" and then showed us in the calculator.

For homework we were assigned to try #7 in the review package. Thats right, all you have to do is try and give it your best shot.


P.S. Skew- in statistics skew is the asymmetry of a distribution around its mean. Skew can be positive or negative. So in english this means the unbalanced distribution of the numbers around the average number.

Work Period...

Today Mr. Max was gone... So we had a work period. The sub we had was a very nice lady and she told Mr. Max that we actually worked. Yay us! We were to go to a website called 'seeingstatistics.com' and teach ourselves. Most took notes of the website to keep it fresh in our minds. We had homework due, but the due date was moved to the fifteenth (today)... Overall it was a great day and it was nice to have a period to just work.

So friday we skipped mental math, and went right into the lesson which was measures of central tendancy & variability. The definition of this is simply it answeres the question how close are a bunch of values to the middle values.

Then we went on to talk about mean, median and mode.

Median - The middle number in a given sequence of numbers, taken as the average of the two middle numbers when the sequence has an even amount of numbers

ex) 4 is the median of 1, 3, 4, 8, 9.

Mean

Mode

Here is one of the examples that Mr. Max showed us.

Here are the measures of variability, i was not sure how to explain it.